- Starter Code
- Related Class Materials
- Introduction
- Task 1: Adder Unit
- Task 2: Compare Unit
- Task 3: Boolean Unit
- Task 4: Shifter
- Task 5: Multiplier
- Task 6: Assembling the ALU
- Summary
50.002 Computation Structures
Information Systems Technology and Design
Singapore University of Technology and Design
Lab 3: Arithmetic Logic Unit
Starter Code
Please clone the starter code from this repository, then open it with Alchitry Lab V2.
git clone https://github.com/natalieagus/50002-lab3-alu.git
You will complete 1D Checkoff 1 by completing this Lab 3. Lab 3 spans two weeks: this week and the next. Do not forget to do the regular lab questionnaire individually as well.
Related Class Materials
The lecture notes on Logic Synthesis and Designing an Instruction Set are closely related to this lab.
Design 5 combinational ALU components: adder/subtractor, compare, boolean, shifter, and multiplier
Related sections in the notes: Logic Synthesis
- N-input gates (all kinds of gates to produce the logic of each component in the ALU)
- Special combinational logic devices (multiplexer with 1 or 2 selectors, and combining multiplexers together to form an even bigger one)
- Basics of programmable control systems (using control signals like ALUFN to perform different operations (
ADD,SHIFT,MUL, etc) between two 32-bit inputs A and B in the same ALU circuit – no hardware modification needed).
This lab will deepen your understanding of building circuits to achieve specific logic functions. For instance, an adder circuit performs binary addition on inputs A and B. You’ll also make it programmable using the ALUFN control signal.
You are NOT allowed to use any of Lucid’s math and comparison operators when implementing this lab’s ALU 13 functionalities. This is the requirement of your 1D project because we would like you to learn the basics and not solely rely on Vivado’s capability on creating components of the ALU. Please give the 1D project handout a read for further information.
Please follow the implementation of the units from the given schematics in this lab. For instance, you shouldn’t implement shifts (SHL, SHR, SRA) trivially using
>>and<<, and you should’t implement ADD and SUB using+or-, etc. Instead you should create a ripple carry adder unit to implement ADD/SUB functionality, and create the shifter unit using multiplexers as shown in this handout.Failure to comply will result in -2% of your overall grades. However, you can use them for array indexing or checking conditions in loops.
Introduction
In this lab, we will build a 32-bit arithmetic and logic unit (ALU) for the Beta processor. You will need this for your 1D Project Checkoff 1 and Lab 4 (Building the Beta).
Arithmetic Logic Unit (ALU)
The ALU is a combinational logic device that has two 32-bit inputs (which we will call “A” and “B”) and produces one 35-bit output:
alu[31:0],Z,V, andN. We will start by designing the ALU modularly. It is composed from five as a separate modules, each producing its own 32-bit output. We will then combine these outputs into a single ALU result.
In this lab, we will attempt to create a 32-bit ALU. It is one of the components inside our Beta CPU. We will eventually utilise our work here to build an entire Beta CPU circuit in the next lab (Lab 4).
The Arithmetic Logic Unit (ALU) serves as the central core of the CPU, handling a variety of logical computations. Essential operations that a standard ALU encompasses are:
- An Addition/Subtraction Unit, facilitating elementary addition and subtraction tasks.
- A Comparison Unit, utilized for branching functions.
- A Boolean Unit, dedicated to boolean operations such as XOR, bit masking, and similar tasks.
- A Shifter Unit, instrumental in operations like division or multiplication by 2, as well as segmenting data.
- A Multiplier Unit, specialized in performing multiplication. The design of this unit is more complicated than the rest of the units.
Important: ALUFN != OPCODE
The
ALUFNsignal is NOT Beta CPUOPCODE, despite both being 6-bit long. These two encodings are NOT the same. TheALUFNis used to control the operation of the ALU circuitry, while the Beta CPUOPCODEis used by the Control Unit to control the entire Beta CPU datapath. In Lab 4, you will build a ROM as part of the Control Unit that will translate theOPCODEfield of a currently executed instruction into the appropriateALUFNcontrol signal.
The ALU can perform the following 13 arithmetic operations based on ALUFN signal given as an input:
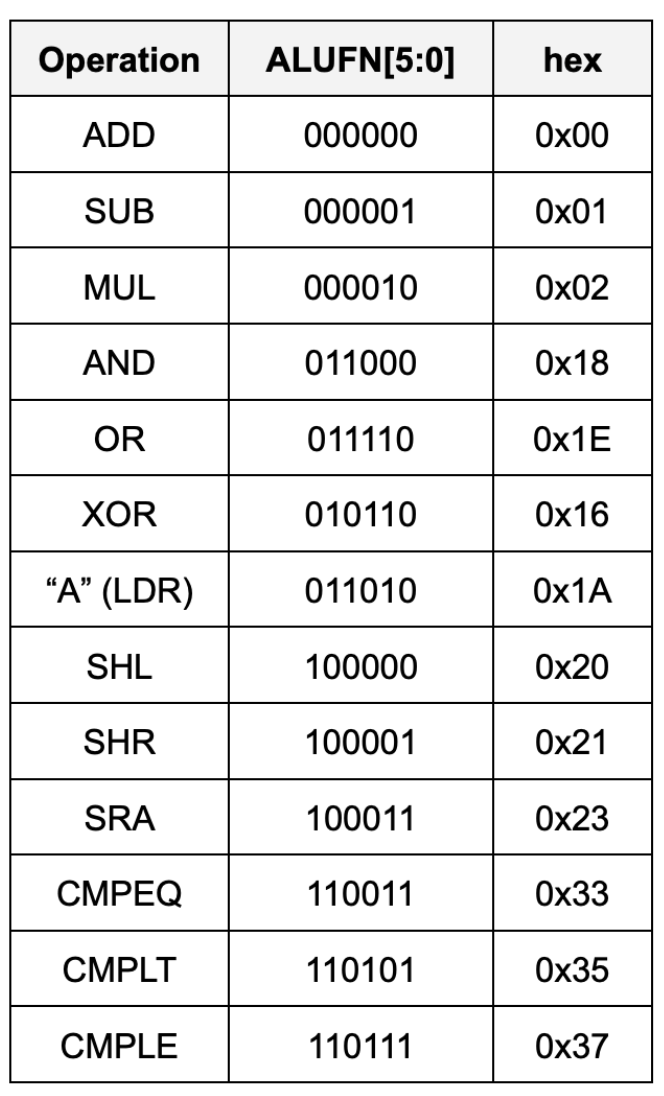
Task 1: Adder Unit
You implemented a simple adder in lab 2 by using Lucid’s arithmetic operator +. You are NOT allowed to do that here and in your 1D project. You need to solely build the components of the ALU using logic units.
Implement an adder/subtractor unit that can add or subtract 32-bit two’s complement (SIGNED) inputs (A[31:0], B[31:0]). It should generate a 35-bit output: (S[31:0]) andZ, V, N signals. A[31:0] and B[31:0] are the 32-bit two’s complement (SIGNED) input operands and S[31:0] is the 32-bit signed output. Z/V/N are the three other output code bits described below:
Zwhich is true when the S outputs are all zero (i.e.,NOR(S) == 1 ? Z = 1 : Z = 0)Vwhich is true when the addition operation overflows (i.e., the result is too large to be represented in 32 bits), andNwhich is true when the S is negative (i.e.,S31 == 1 ? N = 1 : N = 0).
Z, V, N will later be used by the comparator unit (read next section). The following diagram illustrates a suggested implementation of the 32-bit Adder/Subtractor Unit using a Ripple Carry Adder (RCA):
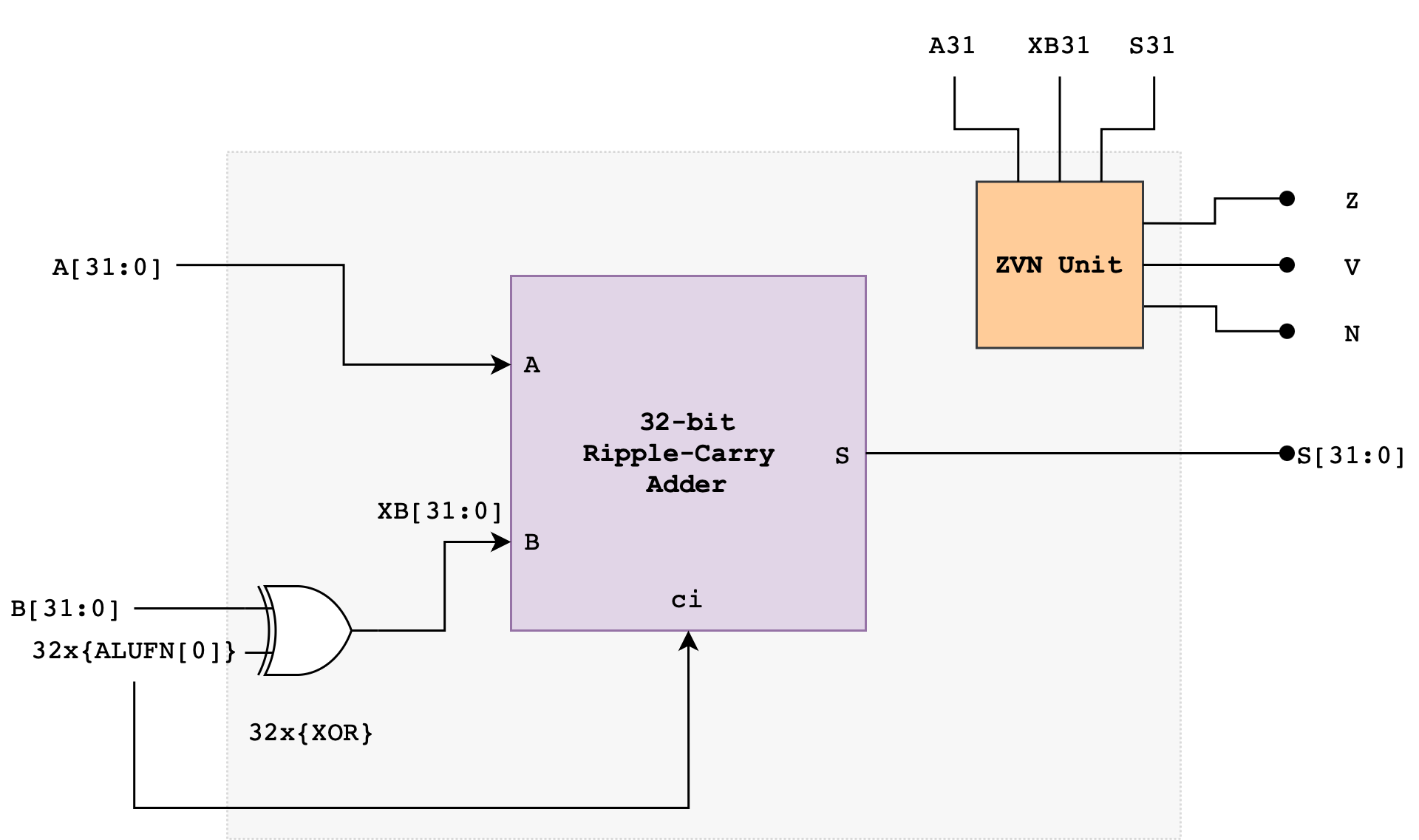
The ALUFN0 input signal controls whether the operation is an ADD or SUBTRACT. ALUFN0 will be set to 0 for an ADD (S = A + B) and 1 for a SUBTRACT (S = A – B). To perform a SUBTRACT, the circuit first computes the two’s complement of the B operand before adding the resulting value with A. The two’s complement of B is computed using the XOR gate and ALUFN0 as carry in to the first Full Adder in the RCA.
The Ripple Carry Adder
A Ripple Carry Adder (RCA) is a simple binary adder that consists of multiple full adders (FA) connected in series. It is used to add two binary numbers.
A full adder (FA) schematic is as shown:

As an exercise, derive the truth table of the FA above.
You can then connect 32 of these in series to form a 32-bit ripple-carry-adder. Below is an example of 4-bit ripple-carry-adder for your reference:

You are encouraged to create the ripple carry adder as a standalone module that accepts the number of bits of addition supported as the parameter.
Computing Overflow: V
Note that overflow can never occur when the two operands to the addition have different signs. If the two operands have the same sign, then overflow can be detected if the sign of the result differs from the sign of the operands. Note that we use XB, not B.
Computing Overflow
VWhy is
Vcomputed like the above? Start by having a small example, let’s say a 4-bit RCA. If we haveA: 0111, andB: 0001, adding both values will result in a positive overflow. The true answer to this should be decimal8. With signed devices, we need 5 bits to represent decimal 8:01000. However since our RCA can only output 4-bits, we have our output as just1000, and this means decimal -8 in a signed 4-bit output. Now think about other possible overflow cases (negative overflow, etc).
Detailed Adder/Subtractor Schematic
Here’s the detailed schematic of the adder to get you started:
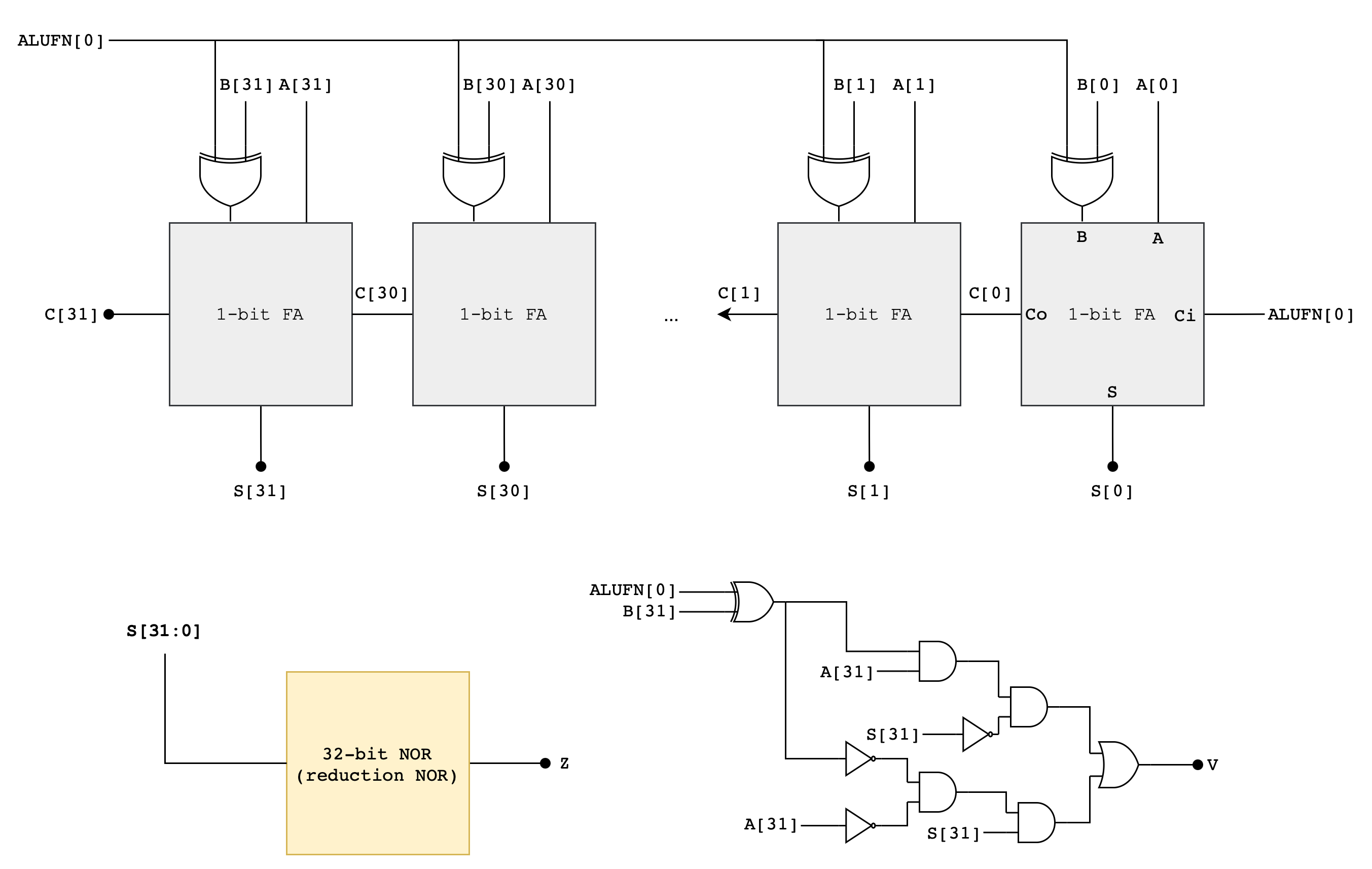
You may start by making a 1-bit Full Adder module first inside fa.luc, and then create a 32-bit RCA module in rca.luc. Afterwards, assemble everything inside adder.luc.
Implementation Tips
Remember that you are NOT allowed to use Lucid’s math operator, such as out = a+b or out = a-b to implement the adder unit. Please follow the implementation of the schematic above. This is part of the requirements of your 1D project. You can however use these operators for indexing.
repeat statement: Recall that the syntax of the repeat statement is as follows:
repeat(i, count, start = 0, step = 1) {
statements
}
You can utilise repeat statement in Lucid V2 (the same applies for Verilog) to duplicate creation of multiple units of fa. For instance, if you have the following fa module interface:

// fa.luc
module fa (
input a,
input b,
input ci,
output co,
output s
)(
// implementation
)
You can utilise it as follows to create a 32-bit Ripple Carry Adder module’s body:
// rca.luc
module rca (
input a[32],
input b[32],
input ci,
output s[32]
) {
fa fa[32];
always {
fa.a = a
fa.b = b
fa.cin = 0;
repeat(i, SIZE){
if (i == 0){
fa.cin[0] = cin
}
else{
fa.cin[i] = fa.cout[i-1]
}
}
s = fa.s; // connect the output bits
}
}
Remember that repeat statement in HDL (Hardware Design Language) like Lucid or Verilog does NOT perform the same way like the for loops we are familiar with in Python or Java. It’s important to understand that in HDLs, repeat loops/statements are used to describe hardware, not to control flow as in software programming. They primarily for generating repetitive hardware structures.
Reduction Operators
In HDLs, reduction operators applies the logic across the bits of the input to produce a single bit output. For instance, given a = 4b1010:
|a(reduction OR) is1|0|1|0, which is1&a(reduction AND) is1&0&1&01which is0^a(reduction XOR) is1^0^1^0which is0
Test
Once you have implemented adder.luc, instantiate and connect its input and output properly in alu.luc so that we can test our adder unit manually.
// alu.luc body
adder.a = a;
adder.b = b;
adder.alufn_signal = alufn_signal;
z = adder.z;
v = adder.v;
n = adder.n;
alu_output = adder.out;
alu_manual_tester
We have instantiated alu in alu_manual_tester for testing. alu_manual_tester is just an IO wrapper connected to alchitry_top so that we can have a more modular structure.
Be organised
You should not implement any logic in
alchitry_top, only I/O connections.
Use io_dip and/or io_button to key in arbitrary values of a, b and alufn, then observe the output at io_led and/or led. Utilise the knowledge you got from lab 2 to thoroughly test your adder before proceeding. If in doubt, consult your instructors/TAs or post questions on edstem.
Tester design suggestion:
- Since
aandbare 32-bit long and we only have 24 dip switches, you can create a tester FSM that allows us to store the first 16 bits ofa, then last 16 bits ofa, then first 16 bits ofb, and finally the last 16 bits ofb. - As for
alufnsignal, you can useio_dip[2][5:0].
Think of useful test cases, such as addition of zeroes, addition of two negative numbers, test overflow, subtraction of -ve values, and so on.
Please be careful with the switches, they’re delicate and easy to break. Use the tip of a male jumper wire to flick them.
Why don’t we just use the operators?
We made it a requirement in your 1D project to NOT use Lucid (or Verilog) math and comparison operators when implementing any of the 13 functionalities of the ALU. You can technically implement a 32-bit adder unit in this manner and let Vivado do the work:
module adder (
input a[32],
input b[32],
input alufn0,
output s[32]
) {
always {
if (alufn0){
s = a + b;
}
else{
s = a - b;
}
}
}
However the above does not allow you to learn anything new. Implementing components of the ALU from scratch has its own benefits as you’re still learning.
Firstly, it enables you to gain a deeper insight into the underlying hardware mechanisms that perform arithmetic operations, and offers a tangible perspective on how abstract mathematical concepts are translated into physical, operational circuits.
This hands-on experience is invaluable for developing an appreciation of the intricacies and challenges associated with digital circuit design, including considerations of timing, power consumption, and scalability.
Secondly, it encourages problem-solving skills, requiring you to apply logic and reasoning to create efficient and functional circuits, along with the first few weeks of 50.002 materials. This process enhances your ability to design, troubleshoot, and optimize digital systems, skills that are crucial for both academic and professional success in the CS field.
Finally, it cultivates an appreciation for the evolution of digital design methodologies and the role of automation in modern engineering. You are not learning how to rely on Vivado but rather to figure out how it works under the hood.
Task 2: Compare Unit
Implement a 32-bit compare unit that generate 1 bit output, depending on the following conditions:
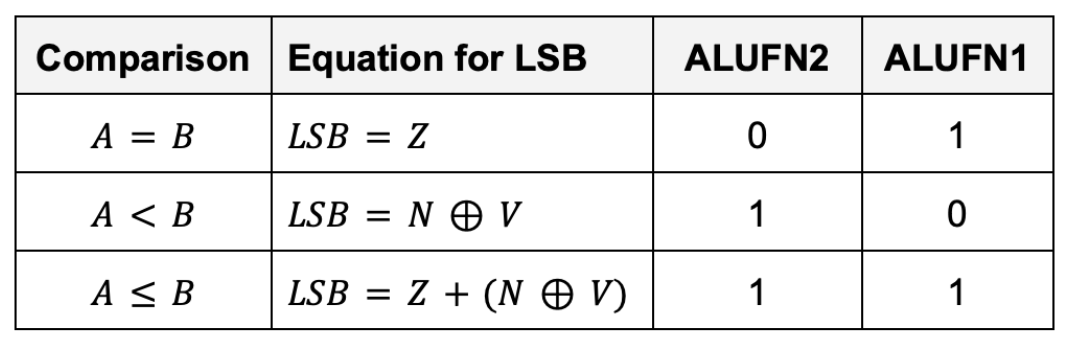
The inputs to the compare unit are:
- The
ALUFNcontrol signals (used to select the comparison to be performed), in particular:ALUFN[2:1] - The
Z,V, andNbits. They’re the output of the adder/subtractor unit. The adder must be in subtraction mode.
Why should the adder be in subtraction mode?
Performance
The Z, V and N inputs to this circuit can only be produced by the adder/subtractor unit. That means we need to first perform a 32-bit addition/subtraction between a and b before we can compare them. This means there’s some significant tpd to produce the output of the compare unit as the RCA is considerably slow.
In real life, you can speed things up considerably by thinking about the relative timing of Z, V and N and then designing your logic to minimize delay paths involving late-arriving signals. For instance, if you need to perform computations involving Z and other variables, you can compute those intermediary output involving the other variables first while “waiting” for Z. We do not need to worry much about it in this Lab as Vivado will do all sorts of optimisation for you.
Detailed Compare Unit Schematic
Here’s the detailed schematic of the compare unit. Pay close attention to the bit selector and the corresponding inputs at the mux:
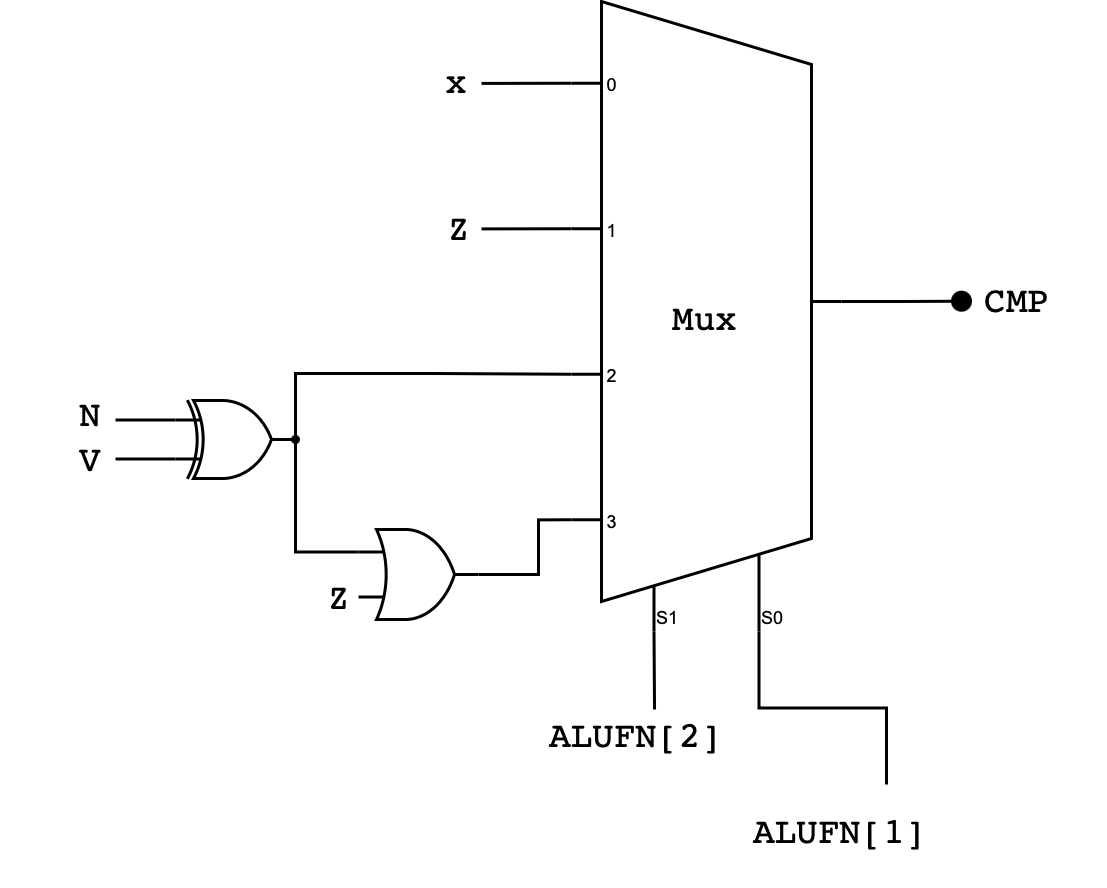
Implementation Tips
Since you are not allowed to use Lucid’s math and comparison operators to implement this lab, it will be beneficial for you if you create a mux_4 unit first:
// mux_4.luc
module mux_4 (
input s0, // selectors
input s1,
input in[4], // inputs
output out
) {
always {
case (c{s1, s0}){
b00: out = in[0];
b01: out = in[1];
b10: out = in[2];
b11: out = in[3];
default:
out = 0;
}
}
}
You can then utilise this inside compare.luc to implement the compare truth table above.
Test
Since the alu must produce a 32-bit output, you should set the higher 31 bits to 0, and set the LSB to the output of the compare unit. You can use concatenation for this.
// alu.luc body
alu_output = c{31x{b0}, compare.cmp}; // concatenation
Your test cases must be comprehensive, and think of possible edge cases such as comparing two negative numbers together, or comparing zeroes.
It is very important to test each of your modules incrementally before proceeding to the next section. Debugging HDL is extremely difficult (no straightforward and convenient print statements or debugger to “pause” execution), so we shall minimise propagation of errors by testing each small module carefully.
Task 3: Boolean Unit
Implement a 32-bit Boolean unit that performs bitwise boolean operation between a and b. The unit should receive 32-bits of a and b as inputs, as well as 4-bit ALUFN[3:0] input, and produce a 32-bit output. In particular it should perform either AND, OR, XOR, or A bitwise boolean operations, depending on the ALUFN signals supplied:
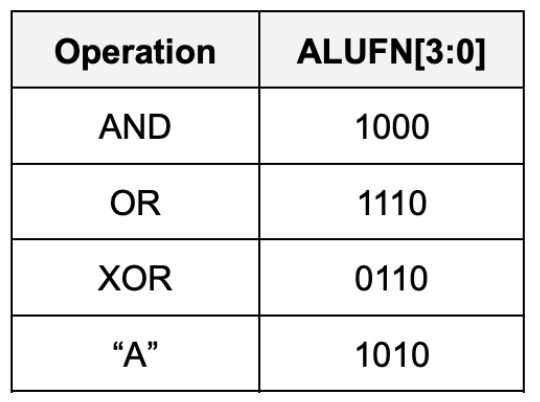
Detailed Boolean Unit Schematic
Here’s the general schematic of the Boolean Unit:

Explanation:
One possible implementation of a 32-bit boolean unit uses 32 copies of a 4-to-1 multiplexer where ALUFN0, ALUFN1, ALUFN2, and ALUFN3 hardcode the operation to be performed, and Ai and Bi are hooked to the multiplexer SELECT inputs. This implementation can produce any of the 16 2-input Boolean functions; but we will only be using 4 of the possibilities: AND, OR, XOR, and A.
In total, you should utilise 32 4-to-1 multiplexers to build the boolean unit. You can utilise the earlier created mux_4.luc module to implement this.
Implementation Tips
You will need 32 copies of ALUFN signals as you will be plugging them into the input ports of each mux_4. To do this, you can use the duplication operator in lucid, for instance:
// boolean.luc
// module declaration
// declaration of modules utilised in boolean unit
mux_4 mux_4_32[32];
always{
// create 32 copies of ALUFN signal as input to each mux_4 unit
// the double curly brackets are intentional because
// we are creating 2D array: 32 by 4 bits
mux_4_32.in = 32x{{alufn_signal[3:0]}};
// the rest of boolean.luc body
}
Test
Please test the boolean.luc module by making appropriate connections in alu.luc before proceeding.
// alu.luc body
alu_output = boolean.bool
Don’t forget to think of useful test cases that test not only the functionality of each boolean operation, but also edge cases (e.g: A and B are all 0 or 1).
Task 4: Shifter
Implement a 32-bit shifter unit that is able to perform a shift left (SHL), shift right (SRA), or shift right arithmetic (SRA) operation on A:
- The
A[31:0]input supplies the data to be shifted - The low-order 5 bits of the
B[4:0]are used as the shift count (i.e., from 0 to 31 bits of shift) - We do not use the high 27 bits of the
Binput (meaning thatB[31:5]is ignored in this unit)
For example, if A: 0x0000 00F0 and we would like to shift A to the left by FOUR bits, the B input should be 0x0000 0004
The desired operation will be encoded on ALUFN[1:0] as follows:

With this encoding, the control signal ALUFN0 controls whether we are performing a left shift or a right shift (SHR). ALUFN1 decides whether we apply the sign extension logic on right shift.
- For
SHLandSHR,0s are shifted into the vacated bit positions. - For
SRA(“shift right arithmetic”), the vacated bit positions are all filled withA31, the sign bit of the original data so that the result will be the same as arithmetically dividing the original data by the appropriate power of 2.
Detailed Shifter Unit Schematic
The simplest implementation is to build three separate shifters: one for shifting left, one for shifting right, and one for shifting right arithmetic.
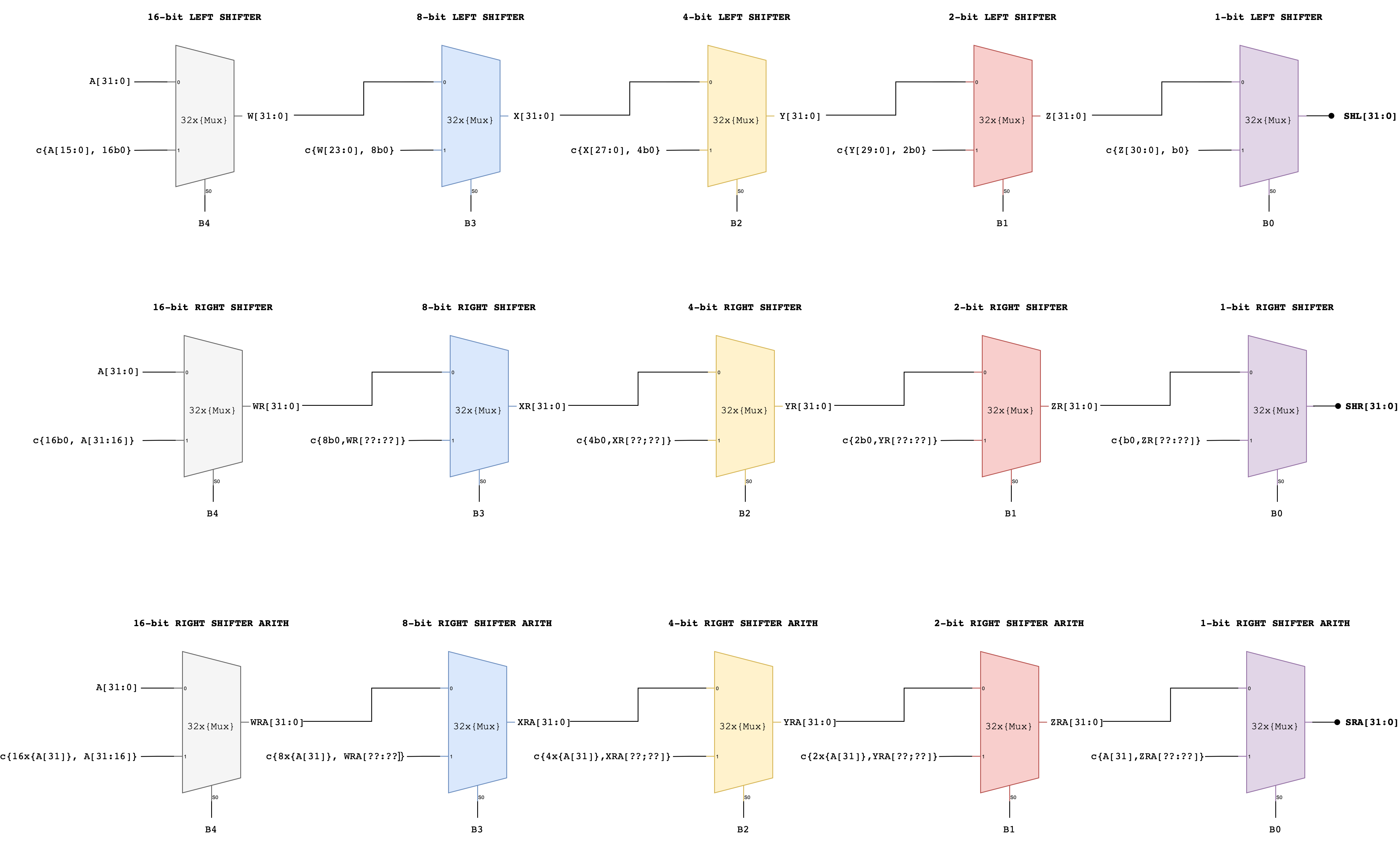
Notice how a multi-bit shift can be accomplished by cascading shifts by various powers of 2.
- For example, a 13-bit shift can be implemented by a shift of 8, followed by a shift of 4, followed by a shift of 1.
- Each shifter unit is just a cascade of multiplexers each controlled by one bit of the shift count.
Afterwards, we can use a 4-way 32-bit multiplexer to select the appropriate answer as the unit’s output.
Alternative Approach: Compact Shifter
Another approach that adds latency but saves gates is to use the left shift logic for both left and right shifts, but for right shifts, reverse the bits of the
Ainput first on the way in and reverse the bits of the output on the way out.Here’s the schematic of this compact shifter.
Implementation Tips
You might want to create a mux_2.luc module here to help your implementation:
module mux_2 (
input s0,
input in[2], // note: you can put input as an array, or declare them separately, e.g: input d0, input d1
// it will affect how you utilise this mux
output out
) {
always {
case (s0) {
0: out = in[0];
1: out = in[1];
default:
out = 0;
}
}
}
Then, you might want to utilise the following x_bit_left_shifter.luc unit, where x is an arbitrary value depending on SIZE parameter. You can supply a SHIFT parameter to this module:
module x_bit_left_shifter #(
// parameter declaration, to be set during module instantiation
// default value given is 8
SHIFT = 8 : SHIFT > -1 & SHIFT < 32,
SIZE = 32 : SIZE > 0
)(
input a[SIZE],
input do_shift,
input pad,
output out[SIZE]
) {
// module declarations
// instantiate mux_2 (32 of them)
// other useful intermediary signals, e.g: shifted_bits[32]
mux2 shift_unit[SIZE];
sig in_1shift_unit[SIZE]; // input s1 of each shift_unit mux
always {
// assign value to shifted_bits[32] depending on the value of SHIFT
// connect the selector of each mux_2 with shift
shift_unit.s0 = SIZEx{do_shift};
in_1shift_unit = c{a[SIZE-1-SHIFT:0], SHIFTx{pad}};
// use a repeat-loop to:
// connect input[0] of each mux_2 with a[i]
// connect input[1] of each mux_2 with the shifted_bits[i]
repeat(i, SIZE){
shift_unit.in[i] = c{in_1shift_unit[i], a[i]};
}
out = shift_unit.out;
}
}
Then you can utilise it to create a left_shifter unit by instantiating 5 of the x_bit_left_shifter:
module left_shifter (
input a[32],
input b[5],
input pad, // 0 or 1 to pad the empty spaces
output shl[32]
) {
// instantiate 5 units of left shifter with different values
x_bit_left_shifter shifter16(#SHIFT(16));
x_bit_left_shifter shifter8(#SHIFT(8));
x_bit_left_shifter shifter4(#SHIFT(4));
x_bit_left_shifter shifter2(#SHIFT(2));
x_bit_left_shifter shifter1(#SHIFT(1));
always {
// connect the padding
shifter16.pad = pad;
shifter8.pad = pad;
shifter4.pad = pad;
shifter2.pad = pad;
shifter1.pad = pad;
// enable or disable each shifter using each bit of b
shifter16.a = a;
shifter16.do_shift = b[4];
shifter8.a = shifter16.out;
shifter8.do_shift = b[3];
shifter4.a = shifter8.out;
shifter4.do_shift = b[2];
shifter2.a = shifter4.out;
shifter2.do_shift = b[1];
shifter1.a = shifter2.out;
shifter1.do_shift = b[0];
// set output as the output of the smallests shifter
shl = shifter1.out;
}
}
Refer to the above two modules to create a right shifter set, or use the alternative approach above.
Instance Parameters
If you want to create instances of N modules with the same parameter, you can use this format:
#PARAM_NAME(VALUE) {
module_type my_module[N]
}
In the above example, all N instances of module_type will have their parameter, PARAM_NAME set to VALUE (must be a constant).
If you want to assign different parameter to each instance, then you need to create an array of N by M, where M is the number of bits required to set VALUE for each instance. For example, we can instantiate 10 my_module with parameter of 8 bits each as follows:
module_type my_module[10](#PARAM_NAME({8d0, 8d1, 8d2, 8d3, 8d4, 8d5, 8d6, 8d7}))
This does not apply to the dff. The clk and rst inputs are always 1 bit and the INIT parameter always applies to the FULL dff. If you do dff storage[32](#INIT(32hABCDFFFF)) then it will store 32-bit value 0xABCDFFFF in these 32 bits dff, where each dff holds 1 bit.
Test
Please test the shifter.luc module by making appropriate connections in alu.luc before proceeding. Be mindful when testing this unit, it should be as comprehensive as the tests you’ve done for the other 3 units above.
// alu.luc body
alu_output = shifter.shift
Task 5: Multiplier
The multiplier unit performs a multiplication between 32-bit inputs A and B each, and produce a 32-bit output.
Multiplying two 32-bit numbers produces a 64-bit product in practice, but the result we’re looking for is just the low-order 32-bits of the 64-bit product.
4-bit Multiplication Logic
It’s hard to imagine a 32-bit multiplier straight up, so let’s scale down to a 4-bit version.
Suppose we want to multiply two 4-bit binary numbers: A = 1011 (which is 11 in decimal) and B = 1101 (which is 13 in decimal).
The multiplication process involves the following steps:
- Write Down the Multiplicands: Write A and B such that each bit of B is aligned under each bit of A.
- Multiply Each Bit of B by A: Multiply each bit of B with the entire number A, shifting the result to the left for each subsequent bit. In binary multiplication, this means we either take A (if the bit in B is 1) or take 0 (if the bit in B is 0).
- Add the Partial Products: Add all the partial products together to get the final result.
1011 (A = 11 in decimal)
x 1101 (B = 13 in decimal)
------
1011 (This is A * 1; the rightmost bit in B is 1)
0000 (This is A * 0; shift left by 1 because we're on the second bit from the right in B)
1011 (This is A * 1; shift left by 2)
1011 (This is A * 1; shift left by 3)
------
10001111 (Sum of the above partial products)
Here is a detailed bit-level description of how a 4-bit by 4-bit unsigned multiplication works. This diagram assumes we only want the low-order 4 bits of the 8-bit product.
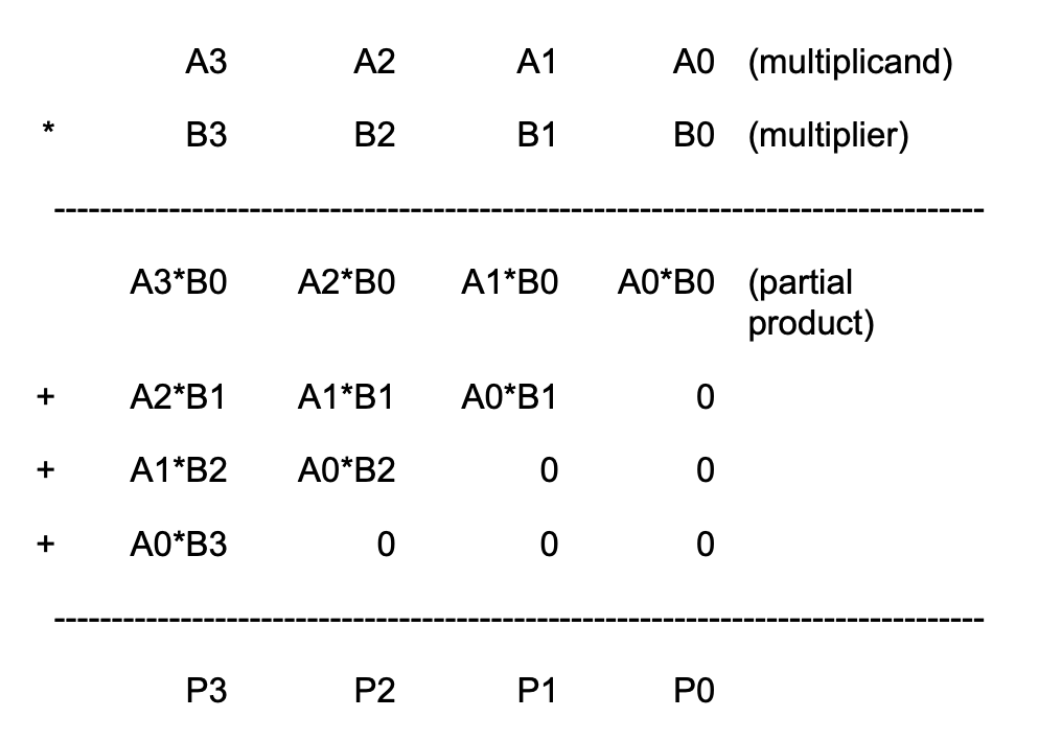
This diagram can be extended in a straightforward way to 32-bit by 32-bit multiplication. Remember that since our machine is only 32-bit, that means we only can store the low-order 32-bits of the result, we don’t need to include the circuitry that generates the rest of the 64-bit product.
4-bit Multiplier Schematic
As you can see from the diagram above, forming the partial products is easy. Multiplication of two bits can be implemented using an AND gate. The hard and tedious part is adding up all the partial products (there will be 32 partial products in your circuit).
- One can use FA units hooked up in a ripple-carry configuration to add each partial product to the accumulated sum of the previous partial products (see the diagram below)
- The circuit closely follows the diagram above but omits an FA module if two of its inputs are
0

Multiplier Analysis
The circuit above works with both unsigned operands and signed two’s complement operands.
Why do we ignore the MSB of the operands?
This may seem strange, don’t we have to worry about the most significant bit (MSB) of the operands? With unsigned operands the MSB has a weight of \(2^{MSB}\) (assuming the bits are numbered 0 to MSB) but with signed operands the MSB has a weight of \(-2^{MSB}\).
Doesn’t our circuitry need to take that into account?
Turns out it does, but when we are only saving the lower half of the product, the differences don’t appear. The multiplicand (A in the figure above) can be either unsigned or two’s complement (signed), and the FA circuits will perform correctly in either case.
When the multiplier (B in the figure above) is signed, we should subtract the final partial product instead of adding it.
- But subtraction is the same as adding the negative, and the negative of a two’s complement number can be computed by taking its complement and adding 1.
- When we work this through we see that the low-order bit of the partial product is the same whether positive or negated.
The low-order bit is ALL that we need when saving only the lower half of the product.
If we were building a multiplier that computed the full product, we’d see many differences between a multiplier that handles unsigned operands and one that handles two’s complement (signed) operands, but these differences only affect how the high half of the product is computed.
Example: 4-bit Signed Multiplication
Let’s use a 4-bit example to illustrate why the lower half of the product is the same whether we are dealing with signed or unsigned numbers, especially in the context of two’s complement arithmetic.
Suppose we have A = 0110 (6 in decimal) multiplied by B = 1101 (-3 in decimal, two’s complement).
As Unsigned Numbers, they are:
A = 0110(6 in decimal)B = 1101(13 in decimal, treated as unsigned)
The multiplication (ignoring overflow) of these two numbers is:
0110 (A = 6)
x 1101 (B = 13, as unsigned)
------
0110 (A * 1)
0000 (A * 0, shift left by 1)
0110 (A * 1, shift left by 2)
0110 (A * 1, shift left by 3)
------
1001110 (78 in decimal, unsigned)
However, the multiplication as signed numbers works differently because the last partial product should be negated:
0110 (A = 6)
x 1101 (B = -3, as signed)
------
0110 (A * 1)
0000 (A * 0, shift left by 1)
0110 (A * 1, shift left by 2)
1010000 (A * 1, shift left by 3, we get 0110000 but it should be negated, resulting in 1010000)
------
1101110 (-18 in decimal, signed)
The negation of
0110000is1010000(flip the bits, then add 1).
In both cases, the lower half of the product (1110) is the same. This is because the difference caused by the negative MSB in the two’s complement representation affects only the higher-order bits, which are outside the lower half of the product.
When multiplying two numbers where the sign of one is significant (like in two’s complement), the alterations to the upper bits due to the sign are not reflected in the lower bits. This is why, in certain computational scenarios where only the lower half of the product is of interest, the circuitry can be simplified as it doesn’t need to differentiate between signed and unsigned numbers.
Design Note
Combinational multipliers implemented as described above are pretty slow! There are many design tricks we can use to speed things up – see the appendix on “Computer Arithmetic” in any of the editions of Computer Architecture: A Quantitative Approach by John Hennessy and David Patterson (Morgan Kauffmann publishers).
Test
As usual, please test your multiplier circuit properly before proceeding. Think of useful test cases, such as multiplication by 0, multiplication by -1, and multiplication by two positive numbers.
Connect the output of your multiplier to the output of your alu for now:
// alu.luc body
alu.out = multiplier.mul;
Failed Timing Warning
This 32-bit combinational multiplier, as part of the ALU, may fail to meet the timing specifications for a 100 MHz clock in a sequential device. However this should still produce a working binary and does not affect other parts of your ALU. It might even give the right output for the multiplier (small bit multiplications).
Basically, if your project fails timing, that means your design logic is too slow to keep up with the 100 MHz clock (i.e., 10 ns per cycle). Even if Vivado compiles it, the actual hardware may behave unpredictably. You should lower the clock frequency in the constraint file (e.g., set to 10 MHz) so Vivado checks for more relaxed timing.
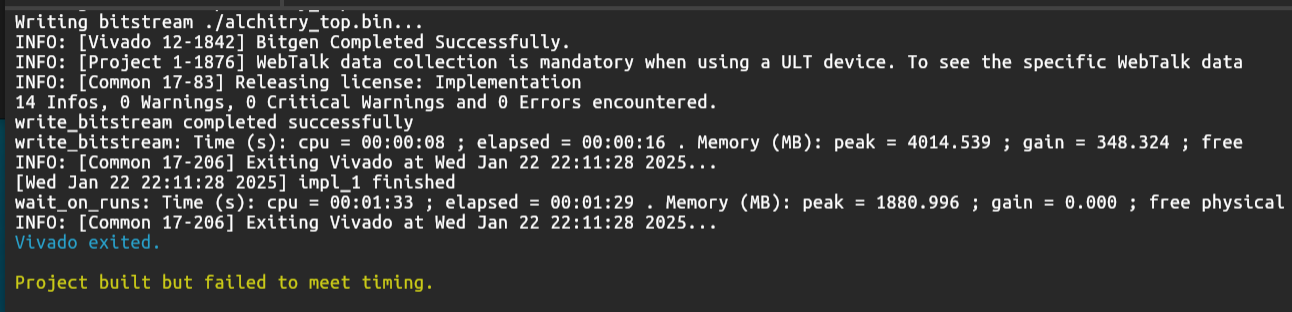
To address this, you can modify the constraint file to let Vivado analyze with a slower clock, e.g 10MHz.
Create a new constraint file (you can name it anything) and paste the content of alchitry.acf to it, and modify the clock signal. Don’t forget to delete the default alchitry.acf.
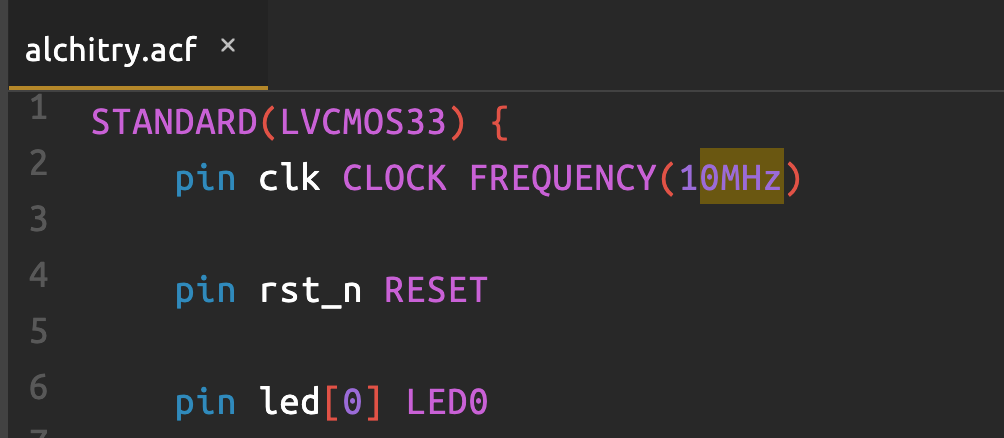
But remember: the onboard clock is still 100 MHz. You must manually slow your logic (e.g., FSM or output updates in ALU Manual Tester) to match this by adding delay logic (like a clock divider). For example: delay each FSM state by 10 times using a counter, so your effective FSM cycle is 1 per microsecond instead of 1 per 10 ns. Consult this handout for details.
As part of your 2D project: Optimisation part, you can consider using other multiplier designs that can pass the original 100MHz clk.
Task 6: Assembling the ALU
You are free to implement each module in whichever way you deem fit, or even come up with a new schematic as long as you don’t use Lucid’s math operators and compare operators to implement any of these 13 functionalities. You can however use them for indexing purposes or conditional loops.
Finally, open alu.luc and assemble the outputs of the finished adder, multiplier, compare, boolean and shift units to produce 32-bit alu output alu_output[31:0] based on the input ALUFN signal. The simplest approach is to use a 4-way 32-bit multiplexer as shown in the schematic below:
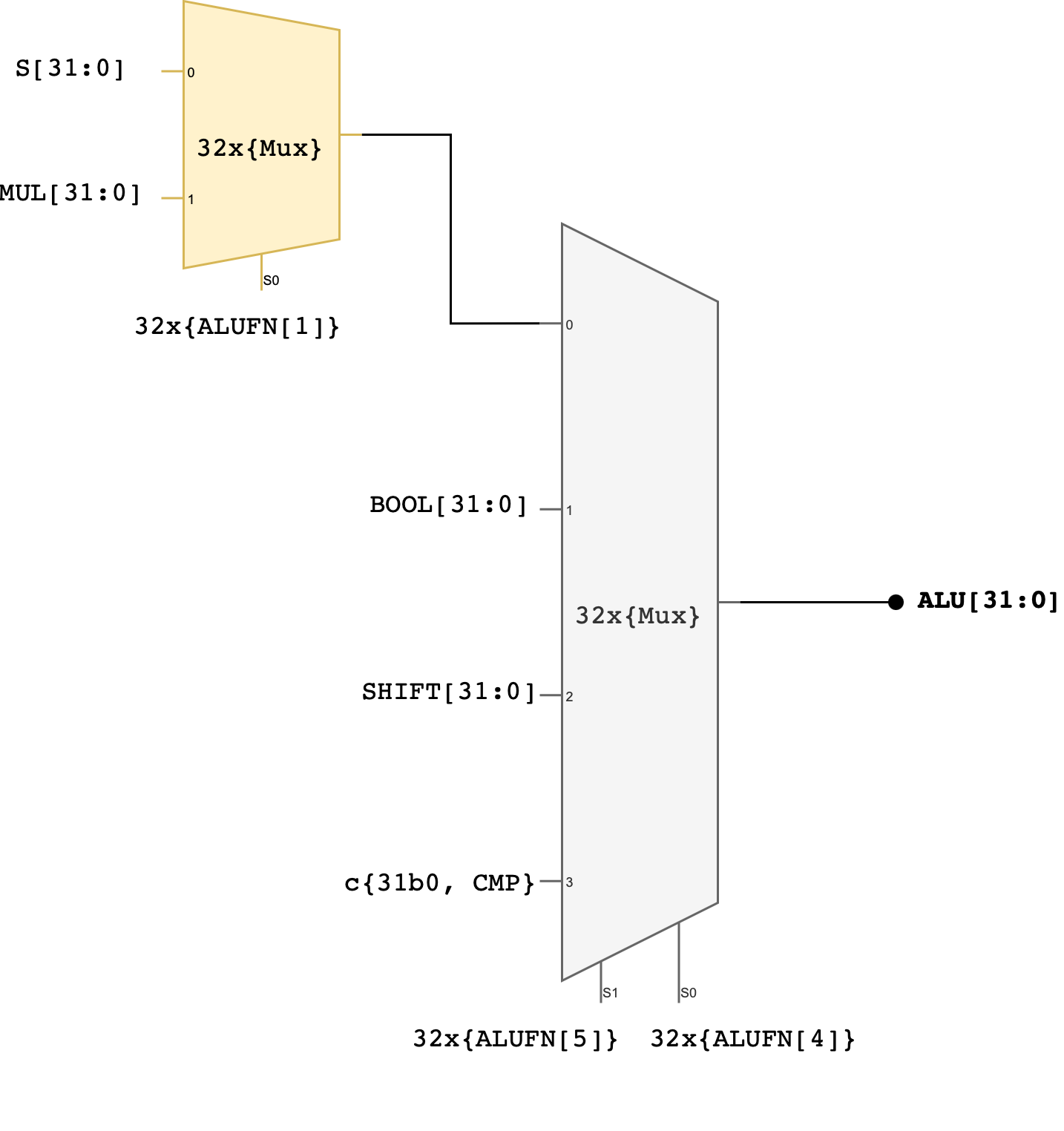
You can use mux_4 above, or use the case statement, or use plain if-else statements.
Two control signals (ALUFN[5:4]) that we have never used before in the individual module have now been utilised to select which unit will supply the value for the ALU output. The encodings for ALUFN[5:0] should follow this table that you’ve seen in the beginning of this handout:

Note that the Z, V, and N signals from the adder/subtractor unit are included in the terminal list for the alu subcircuit (they’re counted as ALU’s output). Please connect these terminals properly in your alu.luc file.
Test
Ensure that you test your ALU comprehensively because you will be using it for your 1D project. You wouldn’t want to discover some bugs down the road as your project grows larger. Here’s some suggestions (you’re not limited to these, think of more!):
- ADD (0x00)
- Zero Addition:
A + 0and0 + Ato ensure correct handling of zero. - Positive Numbers: Add two positive numbers.
- Boundary Values: Add the maximum positive number to itself and ensure correct handling of overflow.
- Check for overflow by adding two very big positive numbers
- Zero Addition:
- SUB (0x01)
- Zero Subtraction:
A - 0and0 - Ato test for correct subtraction with zero. - Underflow: Subtract a larger number from a smaller one to test for underflow.
- Boundary Values: Subtract the maximum positive number from zero and check for correct negative result in two’s complement.
- Zero Subtraction:
- MUL (0x02)
- Zero Multiplication: Multiply by 0 to ensure the result is 0.
- Multiplication by One:
A * 1should yieldA. - Positive Numbers: Multiply two positive numbers and check for correct results.
- Overflow (not compulsory, only if it’s relevant to your project): Multiply two numbers that will cause overflow and ensure it is handled correctly.
- AND (0x18)
- All Zeros and Ones:
A AND 0should be 0,A AND 0xFFFF(assuming 16-bit operands) should beA. - Identity Check:
A AND Ashould giveA. - Complement Check:
A AND NOT Ashould give 0.
- All Zeros and Ones:
- OR (0x1E)
- All Zeros and Ones:
A OR 0should beA,A OR 0xFFFFshould be 0xFFFF. - Identity Check:
A OR Ashould giveA.
- All Zeros and Ones:
- XOR (0x16)
- Identity Check:
A XOR 0should giveA,A XOR Ashould give 0. - Complement Check:
A XOR NOT Ashould give 0xFFFF.
- Identity Check:
- “A” (LDR) (0x1A)
- Load Function: Ensure that inputting
AgivesA, and does not modify it.
- Load Function: Ensure that inputting
- SHL (0x20)
- Zero Shift: Shifting
Aby 0 should yieldA. - Maximum Shift: Shifting a number by the width of the data bus minus one.
- Boundary Cases: Shift a number with a 1 in the MSB and ensure it is handled correctly.
- Zero Shift: Shifting
- SHR (0x21)
- Zero Shift: Shifting
Aby 0 should yieldA. - Maximum Shift: Shifting a number by the width of the data bus minus one.
- Logical Shift: Ensure that the vacated bits are filled with 0.
- Zero Shift: Shifting
- SRA (0x23)
- Arithmetic Right Shift: Ensure the sign bit is replicated to preserve the sign of the number.
- CMPEQ (0x33)
- Equality: Test with equal values to ensure the result is true.
- Inequality: Test with different values to ensure the result is false.
- CMPLT (0x35)
- Less Than: Test where
Ais less thanB. - Greater Than or Equal: Test where
Ais greater than or equal toBto ensure the result is false.
- Less Than: Test where
- CMPLE (0x37)
- Less Than or Equal: Test where
Ais less than or equal toB. - Greater Than: Test where
Ais greater thanBto ensure the result is false.
- Less Than or Equal: Test where
Summary
Congratulations 🎉🎉! You have successfully built a 32-bit ALU in this lab and familiarse yourself with programming FPGA with Lucid. You will be required to utilise it in Lab 4 (Beta CPU), so please keep a copy of your answer.
For your 1D project, you need to demonstrate a working ALU in your Alchitry Au FPGA hardware (not simulator!). You might also want to consider creating automatic tester using the fsm module for your 1D Project Checkoff 1: ALU. Read the FPGA tutorials linked in our course handout for further information, and don’t forget to polish your knowledge on Sequential Logic before proceeding.
Carefully consult Checkoff 1: ALU schedule, requirements and rubrics given in the course handout. Do not miss your checkoff slot.
1D Checkoff 1: ALU
Give the 1D handout a read before Checkoffs to ensure that you don’t miss any information or requirements.
For your Checkoff 1: ALU, you’re also required to create additional functionalities. You are allowed to use Lucid math and comparison operator for this NEW functionality. For example, if your new operation involves ROTATE_SHIFT between A and B, you’re allowed to implement it as follows:
module rotate_shift_right (
input a[32],
input b[5],
output shift[32]
) {
always {
shift = (a >> b) | (in << (32 - b))
}
}
module rotate_shift_left (
input a[32],
input b[5],
output shift[32]
) {
always {
shift = (a << b) | (in >> (32 - b))
}
}
Only the original 13 functionalities must be implemented using logic gates as per the circuitry given in this lab handout.
Avoid Division by Non-Powers of 2
Do not use expressions like a / b where b is not a power of 2.
Division in hardware is complex: it requires a divider circuit, which is large, slow, and significantly more complicated than an adder or shifter. Most hardware division uses restoring or non-restoring algorithms, or iterative subtraction, which take multiple clock cycles. This introduces sequential logic into your ALU, instead of keeping it purely combinational. As a result, it can mess up your timing, increase your critical path delay, and make synthesis and debugging harder.
If you need to divide by a power of 2, use right shifts instead.
When you’re done with the implementation of your ALU, head to eDimension to complete this lab quiz.
 50.002 CS
50.002 CS
